Math and science::Analysis::Tao::07. Series

Geometric series

Let

x

be a real number. If

| x | \geq 1

, then the series

\sum_{n = 0}^{\infty} x^{n}

is divergent. If however

| x | \leq 1

, then the series is absolutely convergent and the sum is given by:

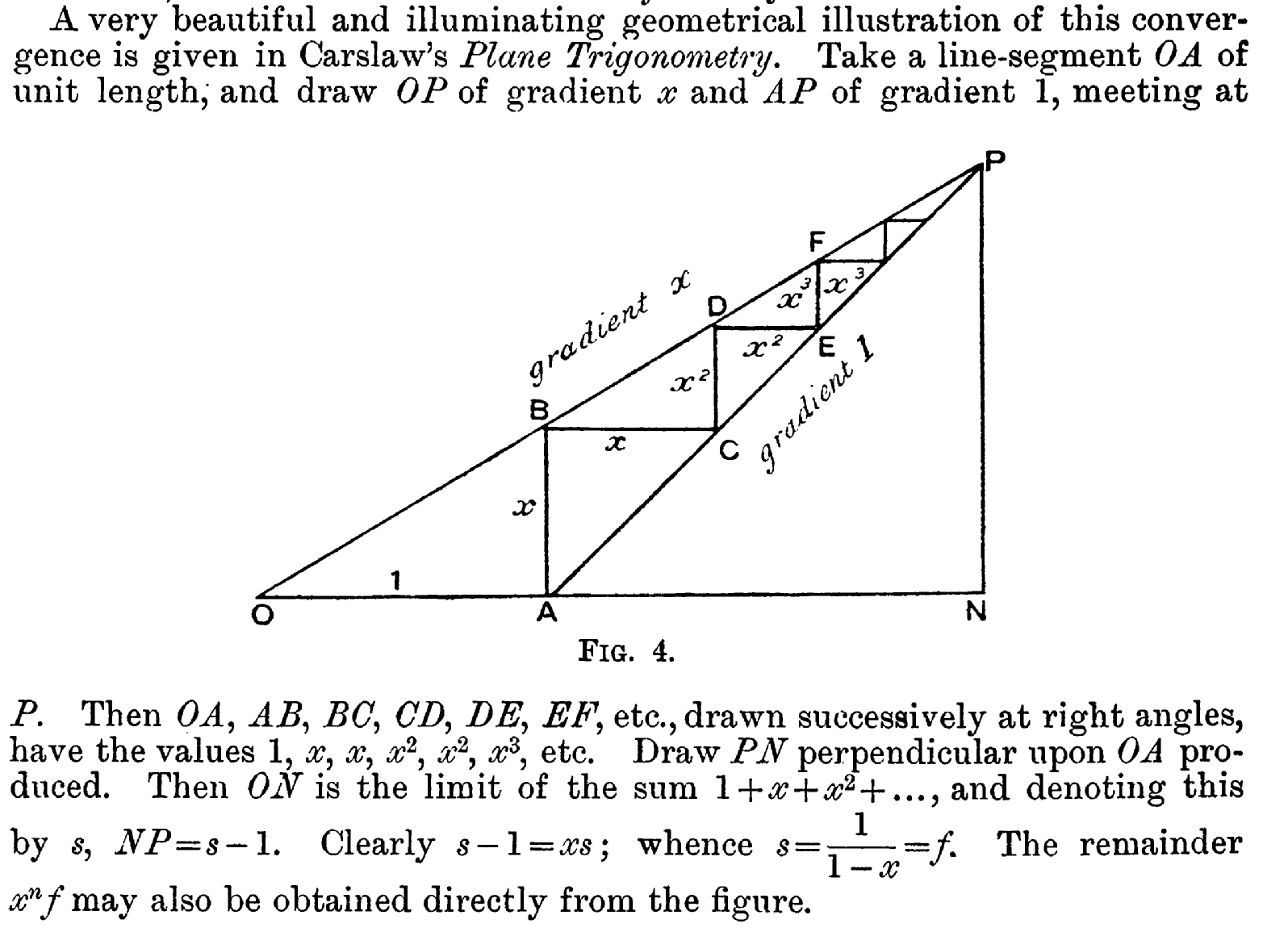
\sum_{n = 0}^{\infty} x^{n} = \frac{1}{1 - x}

On the reverse side, there is some geometric visual reasoning; can you remember what it looks like?

Geometric interpretation

The name "geometric" might have root in whatever led to the geometric mean getting its name. Anayway, below is some nice geometric reasoning. In particular, it shows why when $r \geq 1$ the series will not converge.

To grasp the reasoning, note that with a slope of $x$ , a movement of $1$ horizontally will lead to vertical movement of $x$ , and horizontal movement of $x$ will lead to vertical movement of $x^{2}$ .

Standard proof

The proof of this is is famous and well known

Todo: sort out html video embedding.[anki:play:a:0]

Todo: it would be good to add a proof that addresses all points in the definition/proposition in the style of the book.

28.11.2019