\( \newcommand{\matr}[1] {\mathbf{#1}} \newcommand{\vertbar} {\rule[-1ex]{0.5pt}{2.5ex}} \newcommand{\horzbar} {\rule[.5ex]{2.5ex}{0.5pt}} \)
deepdream of a sidewalk
Show Question
\( \newcommand{\cat}[1] {\mathrm{#1}} \newcommand{\catobj}[1] {\operatorname{Obj}(\mathrm{#1})} \newcommand{\cathom}[1] {\operatorname{Hom}_{\cat{#1}}} \newcommand{\multiBetaReduction}[0] {\twoheadrightarrow_{\beta}} \newcommand{\betaReduction}[0] {\rightarrow_{\beta}} \newcommand{\betaEq}[0] {=_{\beta}} \newcommand{\string}[1] {\texttt{"}\mathtt{#1}\texttt{"}} \newcommand{\symbolq}[1] {\texttt{`}\mathtt{#1}\texttt{'}} \)
Math and science::Analysis::Tao::05. The real numbers

Archimedean property

The Archimedean property is a property of the reals. Roughly, it says: there are no infinitely small or infinitely large elements, when compared to the rationals. It also says that the naturals are not bounded by any real.

Archimedean property

  1. Given any number \( x \in \mathbb{R} \), there exists an \( n \in \mathbb{N} \) satisfying \( n > x \).
  2. Given any real number \( x > 0 \), there exists an \( n \in \mathbb{N} \) satisfying \( \frac{1}{n} < x \).

This is Abbott's description of the Archimedean property.

Tao presents the more common form which goes like:

For any two positive reals \( x \) and \( y \) there exists a natural number \( n \) such that \( ny > x \).

Density of \( \mathbb{Q} \) in \( \mathbb{R} \)

The Archimedean property leads to an important result:

For any reals \( a, b \in \mathbb{R} \) such that \( a < b \) there exists a rational \( q \in \mathbb{Q} \) such that \( a < q < b \).


Proof

The below proof assumes the truth of two statements:

  1. Interspersing of integers by rationals: let \( x \) be a rational number, then there exits an integer \( n \) such that \( n \ge x \ge n+1 \).
  2. Cauchy sequences (sequences of rationals) are bounded (by rationals). Note that a sequence \( (a_n)_{n=1}^{\infty} \) is bounded by \( M \in \mathbb{Q} \) iff \( \forall i \in \mathbb{N}, |a_i| \le M \).

Proof.

  • First prove 1. Let \( x \in \mathbb{R} \). \( x \) is a Cauchy sequence of rationals, and is thus bounded by rationals. Any rational is also bounded by integers, so we have the desired result by transitivity.
  • For part 2, rewrite as \( n > \frac{1}{y} \) and apply part 1.

Density of \(\mathbb{Q} \) in \( \mathbb{R} \), proof.

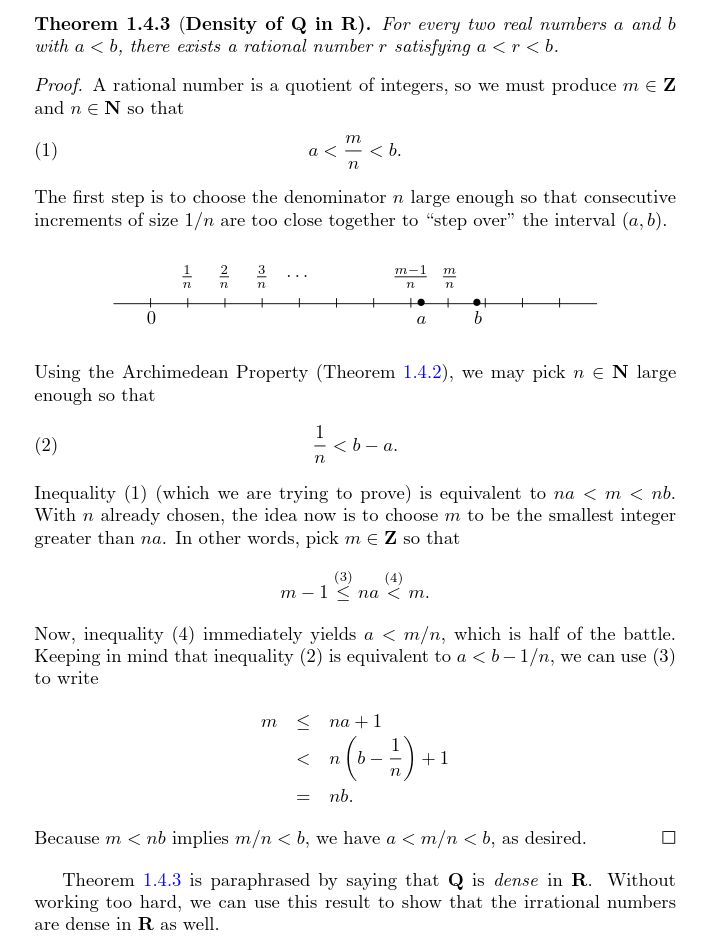
Source

Tao, p114, p115
Abbott, p21