\( \newcommand{\matr}[1] {\mathbf{#1}} \newcommand{\vertbar} {\rule[-1ex]{0.5pt}{2.5ex}} \newcommand{\horzbar} {\rule[.5ex]{2.5ex}{0.5pt}} \)
deepdream of a sidewalk
Show Answer
\( \newcommand{\cat}[1] {\mathrm{#1}} \newcommand{\catobj}[1] {\operatorname{Obj}(\mathrm{#1})} \newcommand{\cathom}[1] {\operatorname{Hom}_{\cat{#1}}} \newcommand{\multiBetaReduction}[0] {\twoheadrightarrow_{\beta}} \newcommand{\betaReduction}[0] {\rightarrow_{\beta}} \newcommand{\betaEq}[0] {=_{\beta}} \newcommand{\string}[1] {\texttt{"}\mathtt{#1}\texttt{"}} \newcommand{\symbolq}[1] {\texttt{`}\mathtt{#1}\texttt{'}} \)
Math and science::Topology

Metric space. Sequence convergence

Sequence convergence, definition

Let \( X \) be a metric space, let \( x_0 \in X \) and let \( (a_n)_{n=1}^{\infty} \) be a sequence in \( X \).

\( (a_n)_{n=1}^{\infty} \) is said to converge to \( x_0 \) iff

[ \( \lim_{?} ? = ? \) ]

[unfolding the definition of limits, this becomes...]



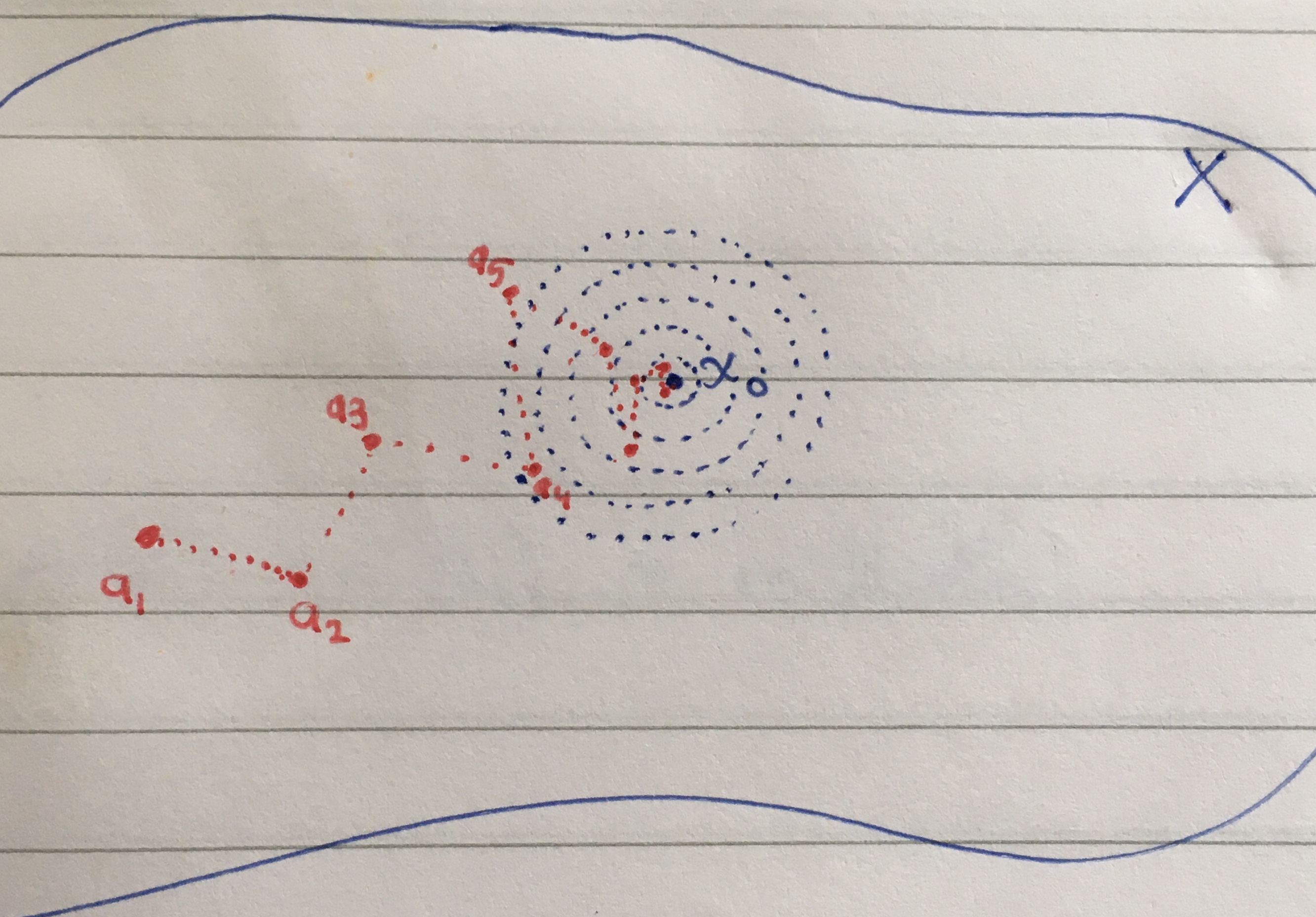
This next result should be internalized. 

Lemma. Closed iff [some property of sequences...]

Let \( X \) be a metric space and \( V \subseteq X \).

Then \( V \) is closed in \( X \) \( \iff \) [some property of sequences...]

The proof was not immediately obvious to me. I did, however, find a way to visualize it, which I think makes the line of reasoning clear. Part of why the proof is important, I think, is that there are only two notions available from which the result needs to be derived: the definition of open sets and the definition of sequence convergence. So, it should be simple, yet it escapes an immediately obvious proof. Thus, I feel there is a mode of thinking that has its essence somewhat distilled into the line of reasoning.