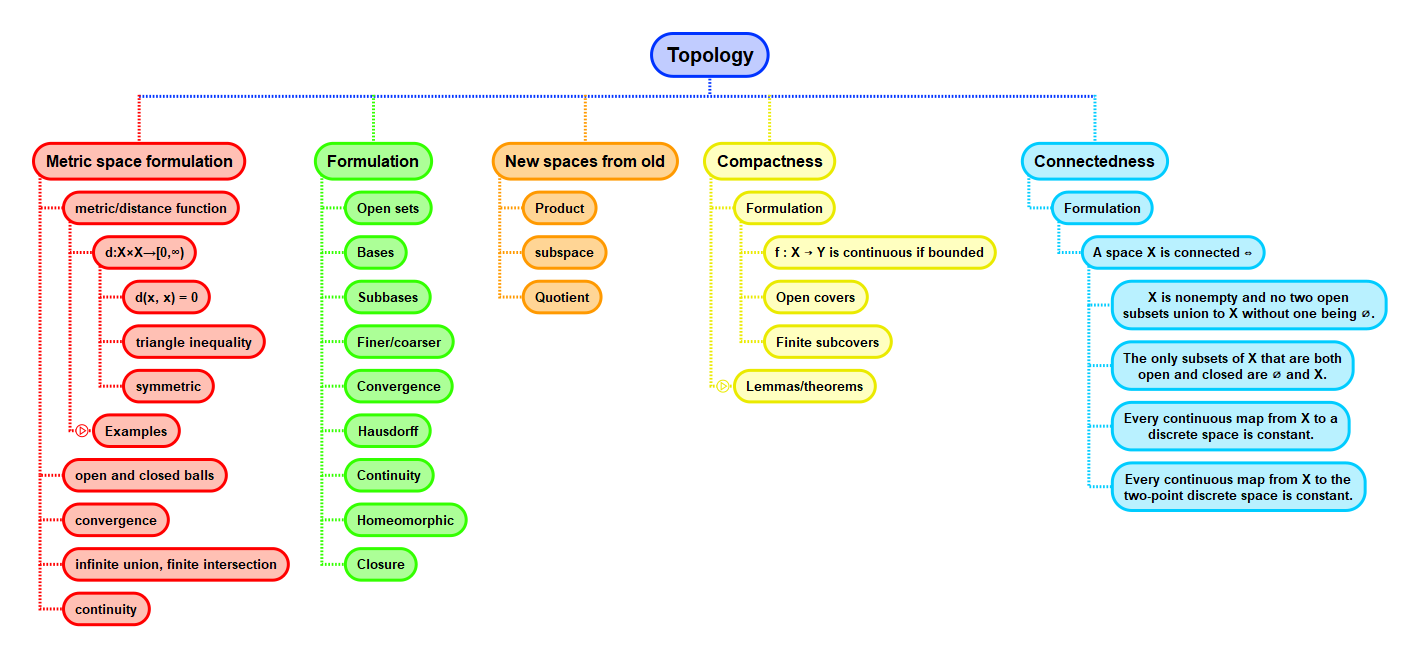
Math and science::Topology
Connectedness Examples
- The space of rationals numbers
- A discrete space with 2 or more points is disconnected. If
- A non-empty indiscrete space is connected.
- An interval topologized as a subspace of
- The space
- The letter 'O' is connected. '0' is a quotient of
- The collection of connected sets in
Context
Source
Munkres, p149Leinster, p69
